Problems

With a non-zero number, the following operations are allowed: \(x \rightarrow \frac{1+x}{x}\), \(x \rightarrow \frac{1-x}{x}\). Is it true that from every non-zero rational number one can obtain each rational number with the help of a finite number of such operations?

Prove that for any positive integer \(n\) the inequality

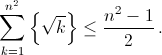
is true.

Calculate \(\int_0^{\pi/2} (\sin^2 (\sin x) + \cos^2 (\cos x))\,dx\).

What has a greater value: \(300!\) or \(100^{300}\)?

Prove that for every natural number \(n > 1\) the equality: \[\lfloor n^{1 / 2}\rfloor + \lfloor n^{1/ 3}\rfloor + \dots + \lfloor n^{1 / n}\rfloor = \lfloor \log_{2}n\rfloor + \lfloor \log_{3}n\rfloor + \dots + \lfloor \log_{n}n\rfloor\] is satisfied.

A numerical sequence is defined by the following conditions: \[a_1 = 1, \quad a_{n+1} = a_n + \lfloor \sqrt{a_n}\rfloor .\]

Prove that among the terms of this sequence there are an infinite number of complete squares.

Orcs and goblins, 40 creatures altogether, are standing in a rectangular formation of \(4\) rows and \(10\) columns. Is it possible that the total number of orcs in each row is \(7\), while the number of orcs in each column is the same?

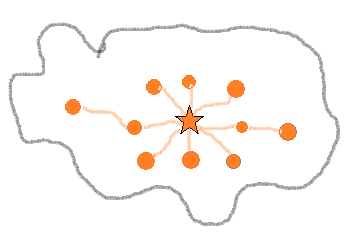
There are some cannons in every fortress on the Cannon Island. The star marks the Grand Fortress, the capital, and the 10 circles mark 10 smaller fortresses. The total number of cannons located in all the fortresses along the east-west road is known to be \(130\). The total number of cannons along each of the other 3 roads is \(80\). Also it is known that there is a total of \(280\) cannons in all the fortresses. How many cannons are in the capital?

There are \(5\) directors of \(5\) banks sitting at the round table. Some of these banks have a negative balance (they owe more money that they have) and some have a positive balance (they have more money that they owe). It is known that for any 3 directors sitting next to each otehr, their 3 banks together have a positive balance. Does it mean that the \(5\) banks together have a positive balance?

A group of Martians and a group of Venusians got together for an important talk. At the start of the meeting, each Martian shook hands with 6 different Venusians, and each Venusian shook hands with 8 different Martians. It is known that 24 Martians took part in the meeting. How large was the delegation for Venus?