Problems
The digits of a 3 digit number \(A\) were written in reverse order and this is the number \(B\). Is it possible to find a value of \(A\) such that the sum of \(A\) and \(B\) has only odd numbers as its digits?
Let \(x\) be a 2 digit number. Let \(A\), \(B\) be the first (tens) and second (units) digits of \(x\), respectively. Suppose \(A\) is twice as large as \(B\). If we add the square of \(A\) to \(x\) then we get the square of a certain whole number. Find the value of \(x\).
Write in terms of prime factors the numbers 111, 1111, 11111, 111111, 1111111.
Specify any solution of the puzzle: \(2014 + YES =BEAR\).
To a certain number, we add the sum of its digits and the answer we get is 2014. Give an example of such a number.
It is known that \(AA + A = XYZ\). What is the last digit of the product: \(B \times C \times D \times D \times C \times E \times F \times G\) (where different letters denote different digits, identical letters denote identical digits)?
The pupils of class 5A had a total of 2015 pencils. One of them lost a box with five pencils, and instead bought a box with 50 pencils. How many pencils do the pupils of class 5A now have?
In one box, there are two pies with mushrooms, in another box there are two with cherries and in the third one, there is one with mushrooms and one with cherries. The pies look and weigh the same, so it’s not known what is in each one. The grandson needs to take one pie to school. The grandmother wants to give him a pie with cherries, but she is confused herself and can only determine the filling by breaking the pie, but the grandson does not want a broken pie, he wants a whole one.
a) Show that the grandmother can act so that the probability of giving the grandson a whole pie with cherries will be equal to \(2/3\).
b) Is there a strategy in which the probability of giving the grandson a whole pie with cherries is higher than \(2/3\)?
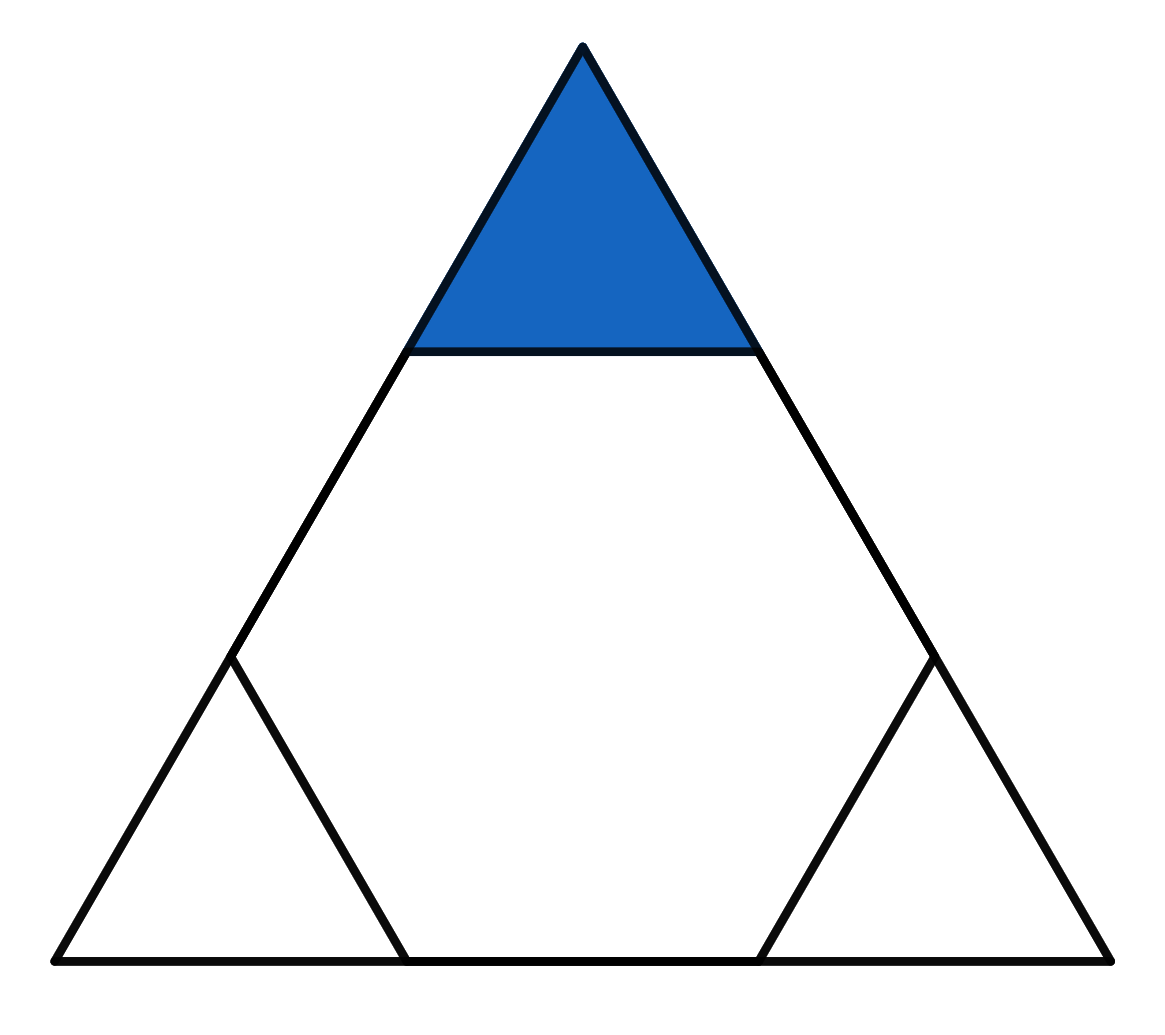
The triangle visible in the picture is equilateral. The hexagon inside is a regular hexagon. If the area of the whole big triangle is \(18\), find the area of the small blue triangle.
Izzy wrote a correct equality on the board: \(35 + 10 - 41 = 42 + 12 - 50\), and then subtracted 4 from both parts: \(35 + 10 - 45 = 42 + 12 - 54\). She noticed that on the left hand side of the equation all of the numbers are divisible by 5, and on the right hand side by 6. Then she took 5 outside of the brackets on the left hand side and 6 on the right hand side and got \(5(7 + 2 - 9)4 = 6(7 + 2 - 9)\). Having simplified both sides by a common multiplier, Izzy found that \(5 = 6\). Where did she go wrong?