Problems
There are \(16\) cities in the kingdom. Prove that it is possible to build a system of roads in such a way that one can get from any city to any other without passing through more than one city on the way, and with at most five roads coming out of each city.
A graph is a finite set of points, some of which are connected with line segments. The points of a graph are called vertices. The line segments are called edges. In this problem set we only consider graphs in which every pair of vertices is connected with one or zero edges.
In a mathematical problem, one may use vertices of a graph to represent objects in the problem, i.e. people, cities, airports, and edges of the graph represent relations between the objects such as mutual friendship, railways between cities, plane routes. As you will see in the examples below, representing the initial problem as a graph can considerably simplify the solution.
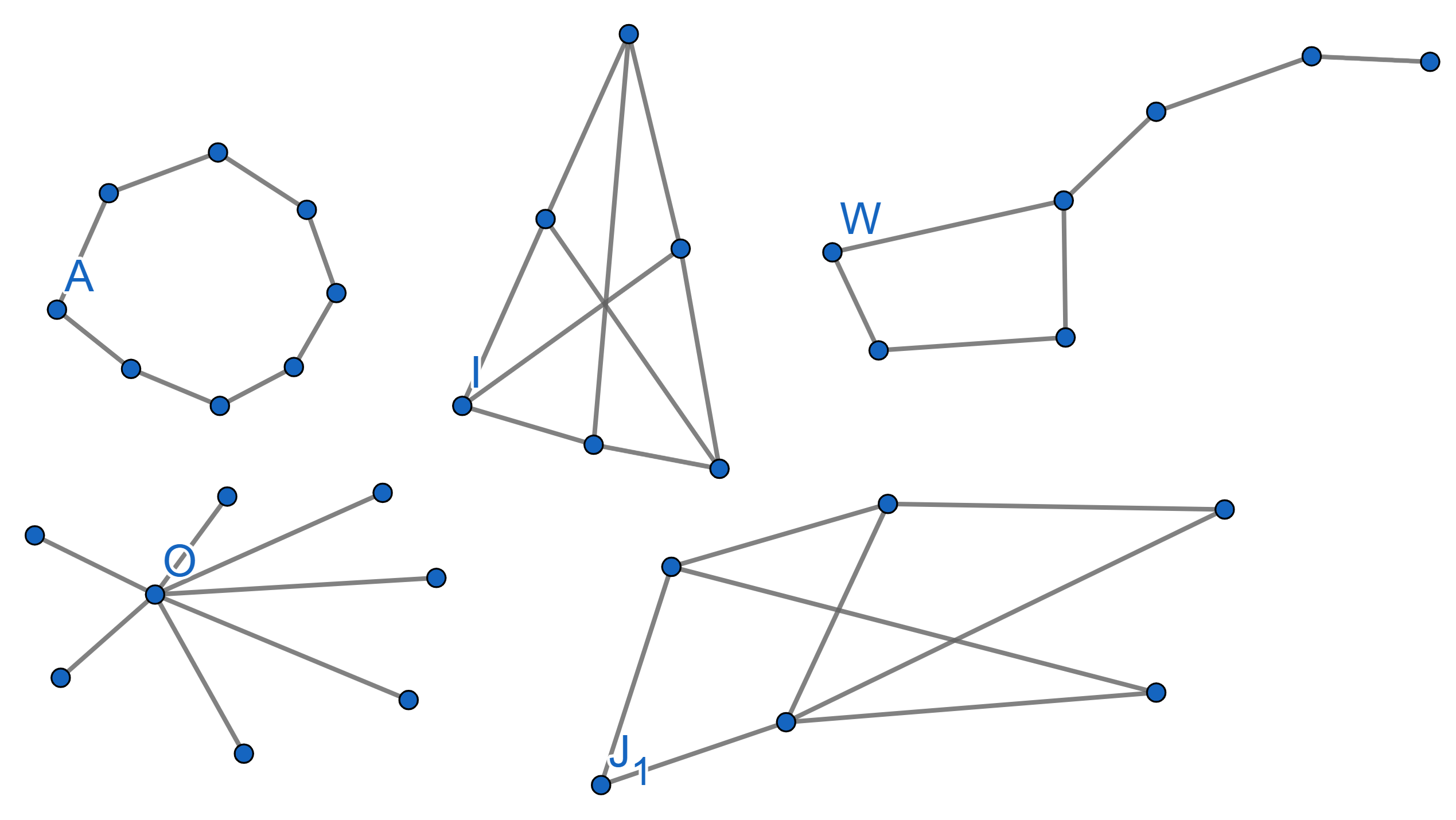
A graph is called Bipartite if it is possible to split all its vertices into two groups in such a way that there are no edges connecting vertices from the same group. Find out whic of the following graphs are bipartite and which are not:
Imagine you are a manager of a very special hotel, a hotel with an infinite number of rooms, where each room has a natural number on the door \(1,2,3,4,...\). Only one guest can stay in each room and in most cases the hotel will be initially full with no vacant rooms left.
You will have to deal with unusual situations that may occur.
Show that a bipartite graph with \(n\) vertices cannot have more than \(\frac{n^2}{4}\) edges.
In a graph \(G\), we call a matching any choice of edges in \(G\) in such a way that all vertices have only one edge among chosen connected to them. A perfect matching is a matching which is arranged on all vertices of the graph.
Let \(G\) be a graph with \(2n\) vertices and all the vertices have degree at least \(n\) (the number of edges exiting the vertex). Prove that one can choose a perfect matching in \(G\).
A new customer comes to the hotel and wants a room. It happened today that all the rooms are occupied. What should you do?
Now imagine you got \(10\) new guests arriving to the completely full hotel. What should you do now?
The next day you have even harder situation: to the hotel, where all the rooms are occupied arrives a bus with infinitely many new customers. In the bus all the seats have numbers \(1,2,3...\) corresponding to all natural numbers. How to deal with this one?
Imagine you have \(2\) new guests arriving to the full hotel. How do you accommodate them?