Problems
Is it possible to colour the cells of a \(3\times 3\) board red and yellow such that there are the same number of red cells and yellow cells?
A coin is tossed six times. How many different sequences of heads and tails can you get?
Eleven people were waiting in line in the rain, each holding an umbrella. They stood closely together, so that the umbrellas of the neighbouring people were touching (see the figure)
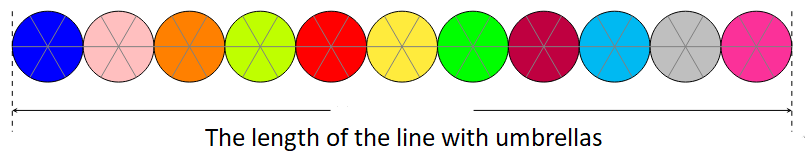
The rain stopped and everyone closed their umbrellas. They then shuffled closer, keeping a distance of \(50\) cm between neighbours. What is the ratio of the old queue length to the new queue length? People can be considered points, and umbrellas are circles with a radius of \(50\) cm.
Cut a square into five triangles in such a way that the area of one of these triangles is equal to the sum of the area of other four triangles.
The prime factorization of the number \(b\) is \(2 \times 5^2 \times 7 \times 13^2 \times 17\). The prime factorization of the number \(c\) is \(2^2 \times 5 \times 7^2 \times 13\). Is the first number divisible by the second one? Is the product of these two numbers, \(b \times c\), divisible by \(49000\)?
A new customer comes to the hotel and wants a room. It happened today that all the rooms are occupied. What should you do?
Now imagine you got \(10\) new guests arriving to the completely full hotel. What should you do now?
Imagine you have \(2\) new guests arriving to the full hotel. How do you accommodate them?
On a \(10\times 10\) board, a bacterium sits in one of the cells. In one move, the bacterium shifts to a cell adjacent to the side (i.e. not diagonal) and divides into two bacteria (both remain in the same new cell). Then, again, one of the bacteria sitting on the board shifts to a new adjacent cell, either horizontally or vertically, and divides into two, and so on. Is it possible for there to be an equal number of bacteria in all cells after several such moves?
Let \(a\), \(b\) and \(c\) be the three side lengths of a triangle. Does there exist a triangle with side lengths \(a+1\), \(b+1\) and \(c+1\)? Does it depend on what \(a\), \(b\) and \(c\) are?