Problems

A game takes place on a squared \(9 \times 9\) piece of checkered paper. Two players play in turns. The first player puts crosses in empty cells, its partner puts noughts. When all the cells are filled, the number of rows and columns in which there are more crosses than zeros is counted, and is denoted by the number \(K\), and the number of rows and columns in which there are more zeros than crosses is denoted by the number \(H\) (18 rows in total). The difference \(B = K - H\) is considered the winnings of the player who goes first. Find a value of B such that

1) the first player can secure a win of no less than \(B\), no matter how the second player played;

2) the second player can always make it so that the first player will receive no more than \(B\), no matter how he plays.

Are there such irrational numbers \(a\) and \(b\) so that \(a > 1\), \(b > 1\), and \(\lfloor a^m\rfloor\) is different from \(\lfloor b^n\rfloor\) for any natural numbers \(m\) and \(n\)?

Two players in turn paint the sides of an \(n\)-gon. The first one can paint the side that borders either zero or two colored sides, the second – the side that borders one painted side. The player who can not make a move loses. At what \(n\) can the second player win, no matter how the first player plays?

Replace the letters with digits in a way that makes the following sum as big as possible: \[SEND +MORE +MONEY.\]

Jane wrote another number on the board. This time it was a two-digit number and again it did not include digit 5. Jane then decided to include it, but the number was written too close to the edge, so she decided to t the 5 in between the two digits. She noticed that the resulting number is 11 times larger than the original. What is the sum of digits of the new number?

a) Find the biggest 6-digit integer number such that each digit, except for the two on the left, is equal to the sum of its two left neighbours.

b) Find the biggest integer number such that each digit, except for the rst two, is equal to the sum of its two left neighbours. (Compared to part (a), we removed the 6-digit number restriction.)

Does there exist an irreducible tiling with \(1\times2\) rectangles of a \(4\times 6\) rectangle?

Irreducibly tile a floor with \(1\times2\) tiles in a room that is a \(5\times8\) rectangle.

Tile the whole plane with the following shapes:

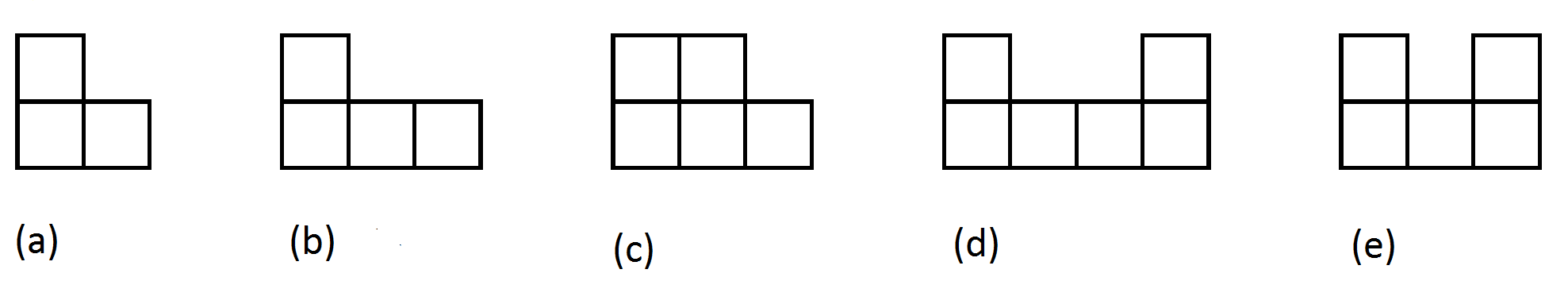
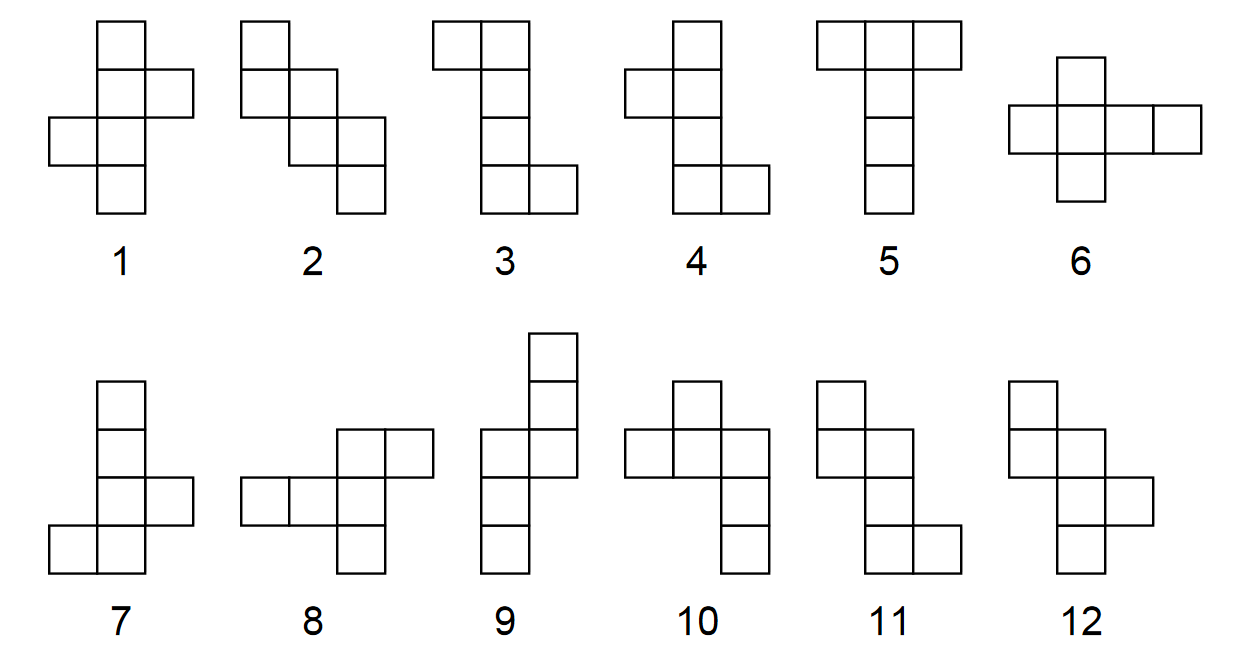
David Smith cut out 12 nets. He claimed that it was possible to make a cube out of each net. Roger Penrose looked at the patterns, and after some considerable thought decided that he was able to make cubes from all the nets except one. Can you figure out which net cannot make a cube?