Problems
Can there exist two functions \(f\) and \(g\) that take only integer values such that for any integer \(x\) the following relations hold:
a) \(f (f (x)) = x\), \(g (g (x)) = x\), \(f (g (x)) > x\), \(g (f (x)) > x\)?
b) \(f (f (x)) < x\), \(g (g (x)) < x\), \(f (g (x)) > x\), \(g (f (x)) > x\)?
Cut the interval \([-1, 1]\) into black and white segments so that the integrals of any a) linear function; b) a square trinomial in white and black segments are equal.
Peter has 28 classmates. Each 2 out of these 28 have a different number of friends in the class. How many friends does Peter have?
\(x_1\) is the real root of the equation \(x^2 + ax + b = 0\), \(x_2\) is the real root of the equation \(x^2 - ax - b = 0\).
Prove that the equation \(x^2 + 2ax + 2b = 0\) has a real root, enclosed between \(x_1\) and \(x_2\). (\(a\) and \(b\) are real numbers).
In the number \(a = 0.12457 \dots\) the \(n\)th digit after the decimal point is equal to the digit to the left of the decimal point in the number. Prove that \(\alpha\) is an irrational number.
With a non-zero number, the following operations are allowed: \(x \rightarrow \frac{1+x}{x}\), \(x \rightarrow \frac{1-x}{x}\). Is it true that from every non-zero rational number one can obtain each rational number with the help of a finite number of such operations?
Find all functions \(f (x)\) defined for all positive \(x\), taking positive values and satisfying the equality \(f (x^y) = f (x)^f (y)\) for any positive \(x\) and \(y\).
The function \(f (x)\) is defined and satisfies the relationship \((x-1) f((x=1)/(x-1)) - f (x) = x\) for all \(x \neq 1\). Find all such functions.
At all rational points of the real line, integers are arranged. Prove that there is a segment such that the sum of the numbers at its ends does not exceed twice the number on its middle.
Prove that for any positive integer \(n\) the inequality
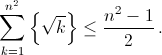
is true.