Problems
There are three piles of rocks: in the first pile there are 10 rocks, 15 in the second pile and 20 in the third pile. In this game (with two players), in one turn a player is allowed to divide one of the piles into two smaller piles. The loser is the one who cannot make a move. Which player would be the winner?
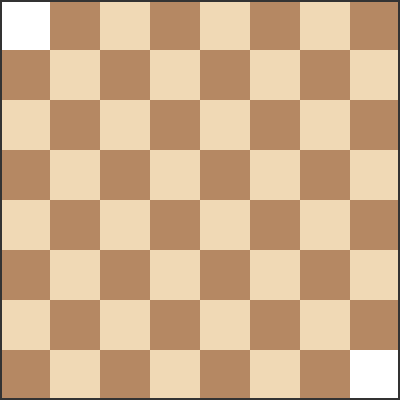
Louise has an \(8\times 8\) chessboard with two opposite corners removes, just like in the picture below. She also has 31 \(2\times1\) dominoes. Can she tile this board with the dominoes she has?
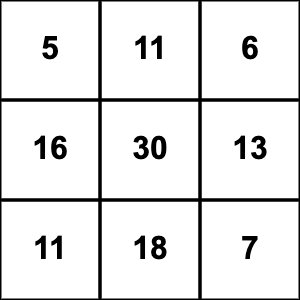
There is a \(3 \times 3\) grid filled with zeros. Louise is allowed to add 1 to each small square inside any \(2\times2\) grid. Can she ever get the following table as a result of her actions?
A rectangular floor is to be covered by \(2 \times 2\) and \(1\times4\) tiles (everything is arranged). Unfortunately one tile got smashed, but we have one more tile of the other kind available. Can we retile the floor perfectly?
a) There are 21 coins on a table with the tails side facing upwards. In one operation, you are allowed to turn over any 20 coins. Is it possible to achieve the arrangement were all coins are facing with the heads side upwards in a few operations?
b) The same question, if there are 20 coins, but you are allowed to turn over 19.