Problems

Find all functions \(f (x)\) defined for all positive \(x\), taking positive values and satisfying the equality \(f (x^y) = f (x)^f (y)\) for any positive \(x\) and \(y\).

Seven triangular pyramids stand on the table. For any three of them, there is a horizontal plane that intersects them along triangles of equal area. Prove that there is a plane intersecting all seven pyramids along triangles of equal area.

Prove that for all \(x\), \(0 < x < \pi /3\), we have the inequality \(\sin 2x + \cos x > 1\).

At all rational points of the real line, integers are arranged. Prove that there is a segment such that the sum of the numbers at its ends does not exceed twice the number on its middle.

Prove that for any positive integer \(n\) the inequality

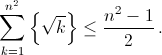
is true.

Find the sum \(1/3 + 2/3 + 2^2/3 + 2^3/3 + \dots + 2^{1000}/3\).

A number set \(M\) contains \(2003\) distinct positive numbers, such that for any three distinct elements \(a, b, c\) in \(M\), the number \(a^2 + bc\) is rational. Prove that we can choose a natural number \(n\) such that for any \(a\) in \(M\) the number \(a\sqrt{n}\) is rational.

A numeric set \(M\) containing 2003 distinct numbers is such that for every two distinct elements \(a, b\) in \(M\), the number \(a^2+ b\sqrt 2\) is rational. Prove that for any \(a\) in \(M\) the number \(q\sqrt 2\) is rational.

Ten pairwise distinct non-zero numbers are such that for each two of them either the sum of these numbers or their product is a rational number.

Prove that the squares of all numbers are rational.

The polynomial \(P (x)\) of degree \(n\) has \(n\) distinct real roots.

What is the largest number of its coefficients that can be equal to zero?